Superposition Theorem in Network Theory

The superposition theorem is a fundamental principle in network theory that applies to linear circuits with multiple independent sources (voltage or current sources). It states that in a linear network containing several independent sources, the voltage across or current through any element in the network can be determined by algebraically summing the contributions from each independent source acting alone while all other independent sources are turned off (replaced by their internal impedances).

Further Explanation

Steps to Apply the Superposition Theorem:

1. Turn Off All Independent Sources Except One:
   • For voltage sources, replace them with a short circuit (i.e., zero voltage).
   • For current sources, replace them with an open circuit (i.e., zero current).
2. Analyze the Circuit: Calculate the voltage across or current through the element of interest with only the one active source.
3. Repeat for Each Independent Source: Repeat the above steps for each independent source in the circuit.
4. Sum the Contributions: Algebraically sum the contributions from each independent source to find the total voltage across or current through the element of interest.

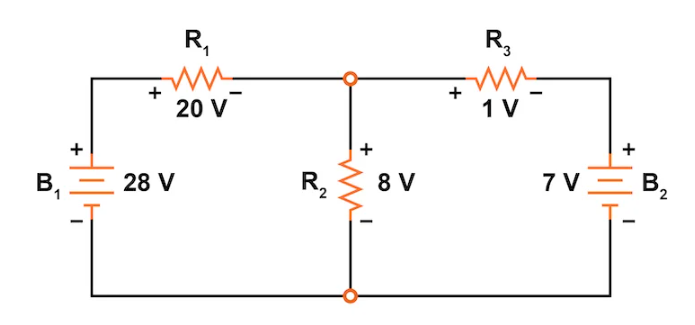
Example:

Consider a simple linear circuit with two independent voltage sources $V_1$ and $V_2$ and resistors $R_1$ and $R_2$ in series.

1. With $V_1$ Active and $V_2$ Turned Off:
   • Replace $V_2$ with a short circuit.
   • Calculate the voltage across or current through each resistor.
2. With $V_2$ Active and $V_1$ Turned Off:
   • Replace $V_1$ with a short circuit.
   • Calculate the voltage across or current through each resistor.
3. Sum the Contributions:
   • Add the voltages or currents obtained from each step to find the total voltage or current.

Key Points:

• Linear Circuits: The superposition theorem only applies to linear circuits, where the parameters (resistance, capacitance, inductance) do not change with voltage or current.
• Independent Sources: The theorem is valid for independent sources. Dependent sources must remain active when other sources are turned off, as their values depend on circuit variables.
• Algebraic Sum: Pay attention to the sign (polarity) of each contribution when summing them.

Benefits:

• Simplifies Analysis: Makes analyzing complex circuits with multiple sources more manageable by breaking the problem into simpler parts.
• Useful in AC Circuits: Can also be applied to AC circuits with sinusoidal sources, considering the phase angles of voltages and currents.

Understanding and applying the superposition theorem is crucial for analyzing and designing linear electrical circuits, providing a systematic approach to determine the effect of each independent source in the network.