Motion in a plane refers to the movement of an object in two dimensions. Unlike one-dimensional motion, where an object moves along a straight line, motion in a plane involves movement along both the x and y axes.
Table of Contents
Types of Motion in a Plane
- Projectile Motion: This is the motion of an object projected into the air with an initial velocity. The object moves under the influence of gravity.
- Circular Motion: This is the motion of an object in a circular path. The object’s distance from a fixed point (the center) remains constant.
Scalar Quantities
A scalar quantity is a physical quantity that is completely described by its magnitude. It does not have a direction associated with it.
Examples of scalar quantities:
- Mass
- Time
- Temperature
- Speed
- Energy
- Distance
Vector Quantities
A vector quantity is a physical quantity that has both magnitude and direction. It is often represented by an arrow, where the length of the arrow represents the magnitude and the direction of the arrow indicates the direction.
Examples of vector quantities:
- Displacement
- Velocity
- Acceleration
- Force
- Momentum
- Electric field
Algebraic Operations on Vectors
Vector Addition
The sum of two vectors is found by adding their corresponding components.
Geometric Representation:
- Triangle Method: Place the tail of the second vector at the head of the first vector. The vector from the tail of the first vector to the head of the second vector is the sum.
- Parallelogram Method: Place both vectors with their tails at the same point. Complete the parallelogram. The diagonal of the parallelogram represents the sum of the vectors.
Algebraic Representation:
If \(\vec{A} = (A_x, A_y, A_z)\) and \(\vec{B} = (B_x, B_y, B_z)\), then their sum \(\vec{C} = \vec{A} + \vec{B}\) is given by:
\[
\vec{C} = (A_x + B_x, A_y + B_y, A_z + B_z)
\]
Vector Subtraction
The difference between two vectors is found by adding the first vector to the negative of the second vector.
Geometric Representation:
- Reverse the direction of the vector to be subtracted.
- Use the triangle method to add the first vector and the reversed vector.
Algebraic Representation:
If \(\vec{A} = (A_x, A_y, A_z)\) and \(\vec{B} = (B_x, B_y, B_z)\), then their difference \(\vec{D} = \vec{A} – \vec{B}\) is given by:
\[
\vec{D} = (A_x – B_x, A_y – B_y, A_z – B_z)
\]
Scalar Multiplication
Multiplying a vector by a scalar changes the magnitude of the vector but not its direction.
Geometric Representation: Scaling a vector lengthens or shortens it while maintaining its direction.
Algebraic Representation:
If \(\vec{A} = (A_x, A_y, A_z)\) and \(k\) is a scalar, then the product \(\vec{B} = k \vec{A}\) is given by:
\[
\vec{B} = (kA_x, kA_y, kA_z)
\]
Dot Product (Scalar Product)
The dot product of two vectors is a scalar that is the product of their magnitudes and the cosine of the angle between them.
Geometric Representation:
\[
\vec{A} \cdot \vec{B} = |\vec{A}| |\vec{B}| \cos\theta
\]
where \(\theta\) is the angle between \(\vec{A}\) and \(\vec{B}\).
Algebraic Representation:
If \(\vec{A} = (A_x, A_y, A_z)\) and \(\vec{B} = (B_x, B_y, B_z)\), then:
\[
\vec{A} \cdot \vec{B} = A_x B_x + A_y B_y + A_z B_z
\]
Cross Product (Vector Product)
The cross product of two vectors is a vector that is perpendicular to the plane containing the original vectors. Its magnitude is the product of their magnitudes and the sine of the angle between them.
Geometric Representation:
\[
|\vec{A} \times \vec{B}| = |\vec{A}| |\vec{B}| \sin\theta
\]
The direction is given by the right-hand rule.
Algebraic Representation:
If \(\vec{A} = (A_x, A_y, A_z)\) and \(\vec{B} = (B_x, B_y, B_z)\), then:
\[
\vec{A} \times \vec{B} = (A_y B_z – A_z B_y, A_z B_x – A_x B_z, A_x B_y – A_y B_x)
\]
Unit Vectors
A unit vector is a vector that has a magnitude of exactly 1. It is used to specify direction only and does not affect the magnitude of the quantity being described.
- Magnitude: The magnitude (or length) of a unit vector is always 1.
\[
|\hat{u}| = 1
\] - Direction: Unit vectors indicate direction. They are often used to describe the direction of other vectors by scaling them with the magnitude of the original vector.
Unit vectors are typically denoted by a lowercase letter with a “hat” (^) symbol. For example, \(\hat{i}\), \(\hat{j}\), and \(\hat{k}\) are standard unit vectors in the Cartesian coordinate system.
Standard Unit Vectors in Cartesian Coordinates:
- \(\hat{i}\) represents the unit vector in the direction of the x-axis.
\[
\hat{i} = (1, 0, 0)
\] - \(\hat{j}\) represents the unit vector in the direction of the y-axis.
\[
\hat{j} = (0, 1, 0)
\] - \(\hat{k}\) represents the unit vector in the direction of the z-axis.
\[
\hat{k} = (0, 0, 1)
\]
Example:
If a vector \(\vec{A}\) is given by:
\[
\vec{A} = 3\hat{i} + 4\hat{j}
\]
The magnitude of \(\vec{A}\) is:
\[
|\vec{A}| = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5
\]
To express \(\vec{A}\) as a unit vector, we divide it by its magnitude:
\[
\hat{A} = \frac{\vec{A}}{|\vec{A}|} = \frac{3\hat{i} + 4\hat{j}}{5} = \left(\frac{3}{5}\hat{i} + \frac{4}{5}\hat{j}\right)
\]
Here, \(\hat{A}\) is the unit vector in the direction of \(\vec{A}\).
Projectile Motion
Projectile motion refers to the motion of an object that is thrown or projected into the air and is subject to only the acceleration due to gravity. The path that the object follows is called its trajectory, which is typically a parabolic shape.
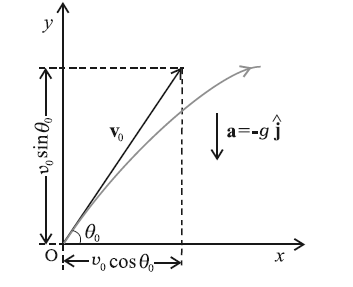
Consider a projectile launched with an initial velocity \( v_0 \) at an angle \( \theta \) with the horizontal. The initial velocity can be resolved into two components:
- Horizontal component: \( v_{0x} = v_0 \cos \theta \)
- Vertical component: \( v_{0y} = v_0 \sin \theta \)
Time of Flight (T)
The time of flight is the total time the projectile remains in the air.
In the vertical direction, the displacement is zero when the projectile returns to the ground level. The vertical motion is influenced by gravity (g), and the equation of motion is:
\[
y = v_{0y} t – \frac{1}{2} g t^2
\]
Setting \( y = 0 \) at the ground level:
\[
0 = v_0 \sin \theta \cdot t – \frac{1}{2} g t^2
\]
Solving for \( t \):
\[
t \left( v_0 \sin \theta – \frac{1}{2} g t \right) = 0
\]
\[
t = 0 \quad \text{or} \quad t = \frac{2 v_0 \sin \theta}{g}
\]
The non-zero solution gives the time of flight:
\[
T = \frac{2 v_0 \sin \theta}{g}
\]
Maximum Height (H)
The maximum height is reached when the vertical component of the velocity becomes zero.
Using the vertical motion equation:
\[
v_y = v_{0y} – g t
\]
At maximum height, \( v_y = 0 \):
\[
0 = v_0 \sin \theta – g t
\]
\[
t = \frac{v_0 \sin \theta}{g}
\]
Now, the vertical displacement at this time (H):
\[
H = v_{0y} t – \frac{1}{2} g t^2
\]
\[
H = v_0 \sin \theta \cdot \frac{v_0 \sin \theta}{g} – \frac{1}{2} g \left( \frac{v_0 \sin \theta}{g} \right)^2
\]
\[
H = \frac{v_0^2 \sin^2 \theta}{g} – \frac{1}{2} \frac{v_0^2 \sin^2 \theta}{g}
\]
\[
H = \frac{v_0^2 \sin^2 \theta}{2g}
\]
Horizontal Range (R)
The horizontal range is the horizontal distance traveled by the projectile during its flight.
Using the horizontal motion equation (with constant velocity \( v_{0x} = v_0 \cos \theta \)):
\[
R = v_{0x} \cdot T
\]
\[
R = v_0 \cos \theta \cdot \frac{2 v_0 \sin \theta}{g}
\]
\[
R = \frac{2 v_0^2 \cos \theta \sin \theta}{g}
\]
Using the trigonometric identity \( 2 \sin \theta \cos \theta = \sin 2\theta \):
\[
R = \frac{v_0^2 \sin 2\theta}{g}
\]
Circular Motion
Circular motion refers to the movement of an object along the circumference of a circle. This motion can be uniform or non-uniform depending on whether the speed of the object remains constant or varies as it moves along the circular path.
Angular Displacement (\(\theta\))
Angular displacement is the angle in radians through which a point or line has been rotated in a specified sense about a specified axis.
For a complete revolution, the angular displacement is \( 2\pi \) radians.
Angular Velocity (\(\omega\))
Angular velocity is the rate of change of angular displacement with respect to time.
\[
\omega = \frac{d\theta}{dt}
\]
For uniform circular motion, \(\omega\) is constant and can be related to the linear speed \( v \) and the radius \( r \) of the circular path by:
\[
\omega = \frac{v}{r}
\]
Centripetal Acceleration (\(a_c\))
Centripetal acceleration is the acceleration directed towards the center of the circular path, causing the change in direction of the velocity vector.
\[
a_c = \frac{v^2}{r} = \omega^2 r
\]
This acceleration is responsible for keeping the object in circular motion.
Centripetal Force (\(F_c\))
Centripetal force is the net force causing the centripetal acceleration of an object in circular motion.
It is directed towards the center of the circle.
\[
F_c = m a_c = m \frac{v^2}{r} = m \omega^2 r
\]
where \( m \) is the mass of the object.
Period (\(T\)) and Frequency (\(f\))
The period \( T \) is the time taken for one complete revolution around the circle.
The frequency \( f \) is the number of revolutions per unit time.
They are related by:
\[
T = \frac{1}{f}
\]
\[
\omega = 2\pi f = \frac{2\pi}{T}
\]
Summary of Important Formulas
- Angular Displacement:
\[
\theta = \frac{s}{r}
\]
where \( s \) is the arc length. - Angular Velocity:
\[
\omega = \frac{d\theta}{dt} = \frac{v}{r}
\] - Centripetal Acceleration:
\[
a_c = \frac{v^2}{r} = \omega^2 r
\] - Centripetal Force:
\[
F_c = m a_c = m \frac{v^2}{r} = m \omega^2 r
\] - Period and Frequency:
\[
T = \frac{1}{f}
\]
\[
\omega = 2\pi f = \frac{2\pi}{T}
\]
Questions
Question 4.1. State, for each of the following physical quantities, if it is a scalar or a vector: volume, mass, speed, acceleration, density, number of moles, velocity, angular frequency, displacement, angular velocity.
Ans:
- Volume – Scalar
- Mass – Scalar
- Speed – Scalar
- Acceleration – Vector
- Density – Scalar
- Number of moles – Scalar
- Velocity – Vector
- Angular frequency – Scalar
- Displacement – Vector
Question 4.2. Pick out the two scalar quantities in the following list:
force, angular momentum, work, current, linear momentum, electric field, average velocity, magnetic moment, relative velocity.
Ans: The two scalar quantities in the list are:
- Work
- Current
Force, angular momentum, linear momentum, electric field, average velocity, magnetic moment, and relative velocity are all vector quantities.
Question 4. 3. Pick out the only vector quantity in the following list:
Temperature, pressure, impulse, time, power, total path length, energy, gravitational potential, coefficient of friction, charge.
Ans: The only vector quantity in the list is: Impulse
Temperature, pressure, time, power, total path length, energy, gravitational potential, coefficient of friction, and charge are all scalar quantities.
Question 4. 4. State with reasons, whether the following algebraic operations with scalar and vector physical quantities are meaningful:
(a) adding any two scalars, (b) adding a scalar to a vector of the same dimensions, (c) multiplying any vector by any scalar, (d) multiplying any two scalars, (e) adding any two vectors, (f) adding a component of a vector to the same vector.
Ans:
(a) Adding any two scalars: No, Scalars can be added if they represent quantities of the same type (e.g., mass + mass, temperature + temperature). The result is another scalar of the same type.
(b) Adding a scalar to a vector of the same dimensions: No, scalars and vectors are fundamentally different types of quantities. A scalar has only magnitude, whereas a vector has both magnitude and direction. You cannot directly add them.
(c) Multiplying any vector by any scalar: Yes, multiplying a vector by a scalar scales the vector’s magnitude by the scalar while retaining the direction. This operation results in a new vector.
(d) Multiplying any two scalars: Yes, scalars can be multiplied together to yield another scalar. This is a standard arithmetic operation.
(e) Adding any two vectors: Yes, Vectors can be added using vector addition, where corresponding components of the vectors are summed. The result is another vector.
(f) Adding a component of a vector to the same vector: Yes, adding a component of a vector to the vector itself is a valid operation.
Question 4.5. Read each statement below carefully and state with reasons, if it is true or false:
(a) The magnitude of a vector is always a scalar.
(b) Each component of a vector is always a scalar.
(c) The total path length is always equal to the magnitude of the displacement vector of a particle.
(d) The average speed of a particle (defined as total path length divided by the time taken to cover the path) is either greater or equal to the magnitude of average velocity of the particle over the same interval of time.
(e) Three vectors not lying in a plane can never add up to give a null vector.
Ans:
(a) The magnitude of a vector is always a scalar.
True. The magnitude of a vector represents its length and does not have direction, making it a scalar quantity.
(b) Each component of a vector is always a scalar.
False. Components of vector are directed in a direction and are vector quantities.
(c) The total path length is always equal to the magnitude of the displacement vector of a particle.
False. The total path length is the actual distance traveled by a particle along its path, while the magnitude of the displacement vector is the shortest straight-line distance between the initial and final positions. The total path length is usually greater than or equal to the displacement magnitude, except in the case of straight-line motion where they are equal.
(d) The average speed of a particle (defined as total path length divided by the time taken to cover the path) is either greater or equal to the magnitude of the average velocity of the particle over the same interval of time.
True. Average speed is the total path length divided by time, while average velocity is the displacement divided by time. Since the total path length is always greater than or equal to the magnitude of displacement, average speed is greater than or equal to the magnitude of average velocity.
(e) Three vectors not lying in a plane can never add up to give a null vector.
True. Three vectors that do not lie in the same plane (i.e., they are not coplanar) cannot form a closed loop, and thus cannot sum to zero. For three vectors to add up to a null vector, they must be coplanar and form a triangle.
Question 4. 6. Establish the following inequalities geometrically or otherwise:
(a) |a+b| ≤ |a| + |b|
(b) |a+b| ≥ ||a| – |b||
(c) |a-b| ≤ |a| + |b|
(d) |a-b| ≥ ||a| – |b||
When does the equality sign above apply?
Answer:
(a) |a+b| ≤ |a| + |b|

- This inequality states that the magnitude of the sum of two vectors is less than or equal to the sum of their magnitudes.
- Geometrically: Consider vectors a and b as two sides of a triangle. The third side is a+b. By the triangle inequality, the length of a+b (its magnitude) is less than or equal to the sum of the lengths of a and b.
- Equality: The equality holds when the vectors a and b are parallel and in the same direction.
(b) |a+b| ≥ ||a| – |b||
- This inequality states that the magnitude of the sum of two vectors is greater than or equal to the absolute difference of their magnitudes.
- Geometrically: This inequality is less intuitive geometrically. However, it can be proved algebraically.
- Equality: The equality holds when the vectors a and b are anti-parallel (opposite directions).
(c) |a-b| ≤ |a| + |b|
- This inequality is similar to (a) with b replaced by -b. Thus, the same geometric interpretation and equality condition apply.
(d) |a-b| ≥ ||a| – |b||
- Similar to (b), with b replaced by -b. The same conditions for equality apply.
Question 4.7. Given vectors a + b + c + d = 0, which of the following statements are correct:
a. Vectors a, b, c, and d must each be a null vector,
b. The magnitude of vectors (a + c) equals the magnitude of vectors(b+ d),
c. The magnitude of a can never be greater than the sum of the magnitudes of b, c, and d,
d. Vectors b + c must lie in the plane of a and d if a and d are not collinear, and in the line of a and d, if they are collinear?
Ans:
(a) Vectors \(\mathbf{a}\), \(\mathbf{b}\), \(\mathbf{c}\), and \(\mathbf{d}\) must each be a null vector:
Incorrect. The equation \(\mathbf{a} + \mathbf{b} + \mathbf{c} + \mathbf{d} = 0\) only requires that the sum of the vectors equals the zero vector. It is possible for each vector to be non-zero as long as their vector sum is zero. For example, \(\mathbf{a} = \mathbf{i}\), \(\mathbf{b} = \mathbf{j}\), \(\mathbf{c} = -\mathbf{i}\), and \(\mathbf{d} = -\mathbf{j}\) satisfy the equation but are not null vectors.
(b) The magnitude of \(\mathbf{a} + \mathbf{c}\) equals the magnitude of \(\mathbf{b} + \mathbf{d}\):
Correct. Given \(\mathbf{a} + \mathbf{b} + \mathbf{c} + \mathbf{d} = 0\), we can rearrange it as \(\mathbf{a} + \mathbf{c} = -(\mathbf{b} + \mathbf{d})\). Taking magnitudes on both sides, we get \(|\mathbf{a} + \mathbf{c}| = |-(\mathbf{b} + \mathbf{d})| = |\mathbf{b} + \mathbf{d}|\), since the magnitude of a vector is always positive.
(c) The magnitude of \(\mathbf{a}\) can never be greater than the sum of the magnitudes of \(\mathbf{b}\), \(\mathbf{c}\), and \(\mathbf{d}\):
Correct. By the triangle inequality, the magnitude of any vector must be less than or equal to the sum of the magnitudes of other vectors whose sum results in the given vector. Given \(\mathbf{a} = -(\mathbf{b} + \mathbf{c} + \mathbf{d})\), we have \(|\mathbf{a}| = |-(\mathbf{b} + \mathbf{c} + \mathbf{d})| = |\mathbf{b} + \mathbf{c} + \mathbf{d}|\).
(d) Vectors \(\mathbf{b} + \mathbf{c}\) must lie in the plane of \(\mathbf{a}\) and \(\mathbf{d}\) if \(\mathbf{a}\) and \(\mathbf{d}\) are not collinear, and in the line of \(\mathbf{a}\) and \(\mathbf{d}\) if they are collinear:
Correct. If \(\mathbf{a}\) and \(\mathbf{d}\) are not collinear, they define a plane. Since \(\mathbf{a} + \mathbf{b} + \mathbf{c} + \mathbf{d} = 0\), the vectors \(\mathbf{b} + \mathbf{c} = -(\mathbf{a} + \mathbf{d})\) lie in the same plane. If \(\mathbf{a}\) and \(\mathbf{d}\) are collinear, then \(\mathbf{b} + \mathbf{c}\) must lie along the line defined by \(\mathbf{a}\) and \(\mathbf{d}\).
Question 4. 8. Three girls skating on a circular ice ground of radius 200 m start from a point P on the edge of the ground and reach a point Q diametrically opposite to P following different paths as shown in Fig. What is the magnitude of the displacement vector for each? For which girl is this equal to the actual length of path skate?
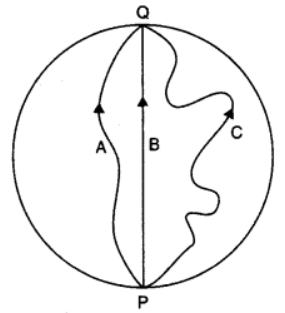
Ans: Magnitude of displacement for each girl is equal to the diameter of the circle.
Diameter = 2 * radius = 2 * 200 m = 400 m
So, the magnitude of the displacement vector for each girl is 400 meters.
For which girl is this equal to the actual length of path skated?
The actual length of the path skated is the distance traveled.
Only girl B takes a path that is a straight line from P to Q, which is the diameter of the circle. Therefore, for girl B, the magnitude of the displacement is equal to the actual length of the path skated.
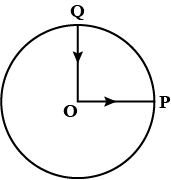
Question 4. 9. A cyclist starts from the centre O of a circular park of radius 1 km, reaches the edge P of the park, then cycles along the circumference, and returns to the centre along QO as shown in Fig. If the round trip takes 10 min, what is the (a) net displacement, (b) average velocity, and (c) average speed of the cyclist?
Ans:
(a) Net Displacement
Displacement is the shortest distance between the initial and final positions of the cyclist. Since the cyclist returns to the center \( O \) after completing the round trip, the initial and final positions are the same.
Net Displacement: \( 0 \) km
(b) Average Velocity
Average velocity is defined as the total displacement divided by the total time taken. Since the net displacement is zero (the cyclist returns to the starting point), the average velocity will also be zero.
Average Velocity: \( 0 \) km/min
(c) Average Speed
Average speed is defined as the total distance traveled divided by the total time taken. Let’s calculate the total distance traveled by the cyclist.
- From \( O \) to \( P \): This is a straight line from the center to the edge of the circular park, which is the radius of the circle.Distance: \( 1 \) km
- Along the Circumference from \( P \) to \( Q \): This is a quarter of the circumference of the circle (since the cyclist travels from one point on the edge to another point that is a quarter of the circle away).Circumference of the circle: \( 2 \pi \times 1 \) km. Quarter circumference: \( \frac{\pi}{2} \) km
- From \( Q \) to \( O \): This is again a straight line from the edge to the center, which is the radius of the circle.Distance: \( 1 \) km
Total Distance Traveled: \( 1 \) km (O to P) + \( \frac{\pi}{2} \) km (P to Q) + \( 1 \) km (Q to O) = \( 2 + \frac{\pi}{2} \) km
Total Time Taken: \( 10 \) min
Average Speed: Total distance traveled / Total time taken
\[
\text{Average Speed} = \frac{2 + \frac{\pi}{2}}{10} \text{ km/min}
\]
Simplifying further:
\[
\text{Average Speed} = \frac{4 + \pi}{20} \text{ km/min} = \frac{4 + \pi}{20} \text{ km/min}
\]
Just convert the above value by putting value of \(\pi\):
\[
\approx 3(4 + 3.14) \text{ km/h} = 21.42 \text{ km/h}
\]
Question 4.10. On an open ground, a motorist follows a track that turns to his left by an angle of 60° after every 500 m. Starting from a given turn, specify the displacement of the motorist at the third, sixth and eighth turn. Compare the magnitude of the displacement with the total path length covered by the motorist in each case.

Solution:
Third Turn (Point D)
Path Analysis:
- From A to B: 500 meters
- From B to C: 500 meters with a 60° turn to the left
- From C to D: 500 meters with another 60° turn to the left
The motorist forms an equilateral triangle AOB.
Displacement (AD): In an equilateral triangle, the displacement from point A to point C is:
\[
AC = 1000 \text{ meters}
\]
Total Path Length: \(3 \times 500 \text{ meters} = 1500 \text{ meters}\)
Sixth Turn (Point A)
Path Analysis: The motorist completes a hexagon ABCDEF.
Displacement: Since the motorist returns to the starting point A:
\[
\text{Displacement} = 0 \text{ meters}
\]
Total Path Length: \(6 \times 500 \text{ meters} = 3000 \text{ meters}\)
Eighth Turn (Point C)
The motorist completes one full hexagon (ABCDEF) and two additional sides (AB, BC).
Displacement (AC): AH is the vector sum of two sides of an equilateral triangle of side 500 m with an angle of 120° between them.
Using the Law of Cosines:
\[
AC = \sqrt{500^2 + 500^2 – 2 \times 500 \times 500 \times \cos(120^\circ)}
\]
\[
AC = \sqrt{500^2 + 500^2 + 500^2} = 500 \sqrt{3} \approx 866 \text{ meters}
\]
Total Path Length: \(8 \times 500 \text{ meters} = 4000 \text{ meters}\)
Comparison
- Third Turn: Magnitude of Displacement: \(1000 \text{ meters}\), Total Path Length: \(1500 \text{ meters}\)
- Sixth Turn: Magnitude of Displacement: \(0 \text{ meters}\), Total Path Length: \(3000 \text{ meters}\)
- Eighth Turn: Magnitude of Displacement: \(866 \text{ meters}\), Total Path Length: \(4000 \text{ meters}\)
Question 4.11. A passenger arriving in a new town wishes to go from the station to a hotel located 10 km away on a straight road from the station. A dishonest cab man takes him along a circuitous path 23 km long and reaches the hotel in 28 min. What is (a) the average speed of the taxi, (b) the magnitude of average velocity? Are the two equal?
Solution:
(a) Average Speed
Average speed = Total distance / Total time
Total distance = 23 km
Total time = 28 minutes = 28/60 hours = 7/15 hours
Average speed = 23 km / (7/15 h) = 49.29 km/h
(b) Average Velocity
Average velocity = Displacement / Total time
Displacement is the straight-line distance between the starting point (station) and the ending point (hotel) = 10 km.
Average velocity = 10 km / (7/15 h) = 21.43 km/h
Are the two equal?
No, the average speed and average velocity are not equal.
Average speed considers the total distance covered, while average velocity considers only the straight-line distance (displacement) between the initial and final points.
Therefore, the average speed of the taxi is 49.29 km/h, the magnitude of the average velocity is 21.43 km/h, and the two are not equal.
Question 4.12. Rain is falling vertically with a speed of 30 m s-1. A woman rides a bicycle with a speed of 10 m s-1 in the north to south direction. What is the direction in which she should hold her umbrella ?
Solution: This problem involves vector addition. The woman’s motion relative to the ground is the vector sum of her velocity and the rain’s velocity.
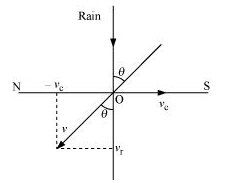
- Rain’s velocity: 30 m/s vertically downward.
- Woman’s velocity: 10 m/s southward.
To protect herself from the rain, the woman must hold her umbrella in the direction of the relative velocity of the rain with respect to her. This relative velocity is the vector difference of the rain’s velocity and the woman’s velocity.
Relative velocity of rain with respect to the woman: This is a vector with a vertical component of 30 m/s downward and a horizontal component of 10 m/s northward (due to the woman’s southward motion).
To find the angle θ the woman should hold her umbrella with respect to the vertical, we can use trigonometry:
- tan θ = horizontal component / vertical component
- tan θ = 10 m/s / 30 m/s = 1/3
- θ = tan⁻¹(1/3) ≈ 18.43°
Therefore, the woman should hold her umbrella at an angle of approximately 18.43° with the vertical, tilted towards the south.
Question 4.13. A man can swim with a speed of 4.0 km/h in still water. How long does he take to cross a river 1.0 km wide if the river flows steadily at 3.0 km/h and he makes his strokes normal to the river current? How far down the river does he go when he reaches the other bank?
Solution:
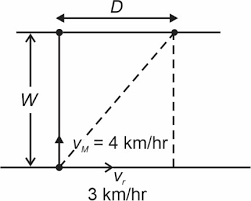
Time to Cross the River
Since the man is swimming normal (perpendicular) to the river current, his swimming speed across the river will be unaffected by the current. The width of the river is given as 1.0 km, and his swimming speed in still water is 4.0 km/h.
The time \( t \) to cross the river can be calculated using the formula:
\[
t = \frac{\text{distance}}{\text{speed}} = \frac{1.0 \text{ km}}{4.0 \text{ km/h}} = 0.25 \text{ hours}
\]
Distance Downstream
While he is swimming across the river, the river current will carry him downstream. The speed of the river current is 3.0 km/h. To find the distance \( d \) he is carried downstream, we use the time calculated above:
\[
d = \text{speed of current} \times \text{time} = 3.0 \text{ km/h} \times 0.25 \text{ hours} = 0.75 \text{ km}
\]